It was a trial. But $40/month is very expensive.



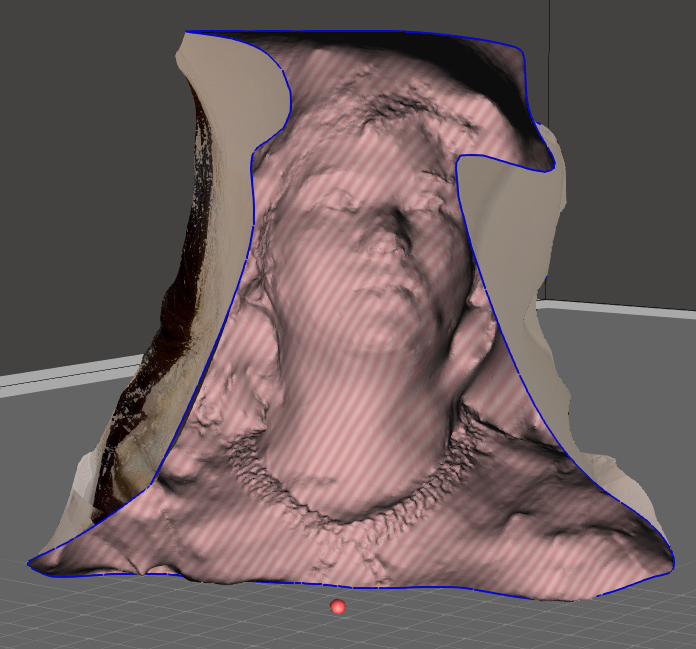

I loaded it up into Autodesk Meshmixer and filled the surface, made it flat and 3d printable.
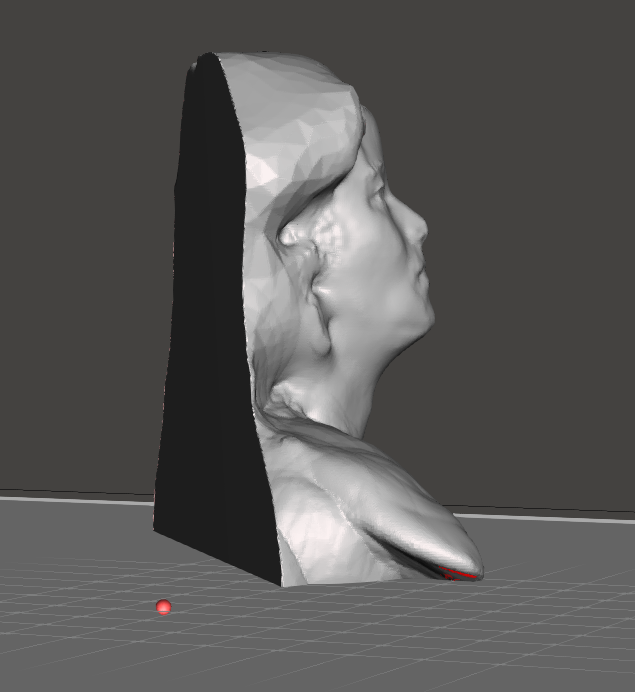
It was a trial. But $40/month is very expensive.





I loaded it up into Autodesk Meshmixer and filled the surface, made it flat and 3d printable.





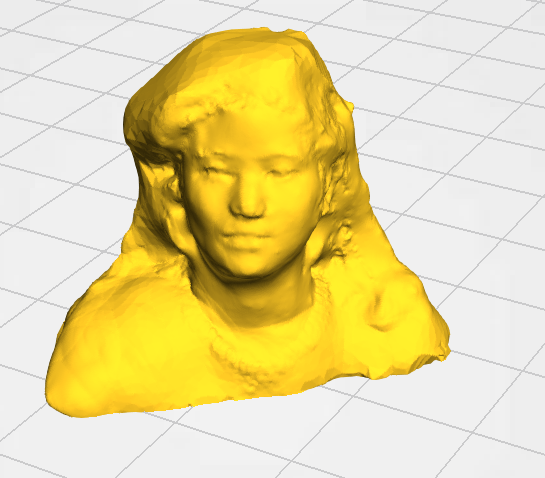
I take aerial lidar point cloud and turn it into a 3D object.
https://www.thingiverse.com/thing:3615141



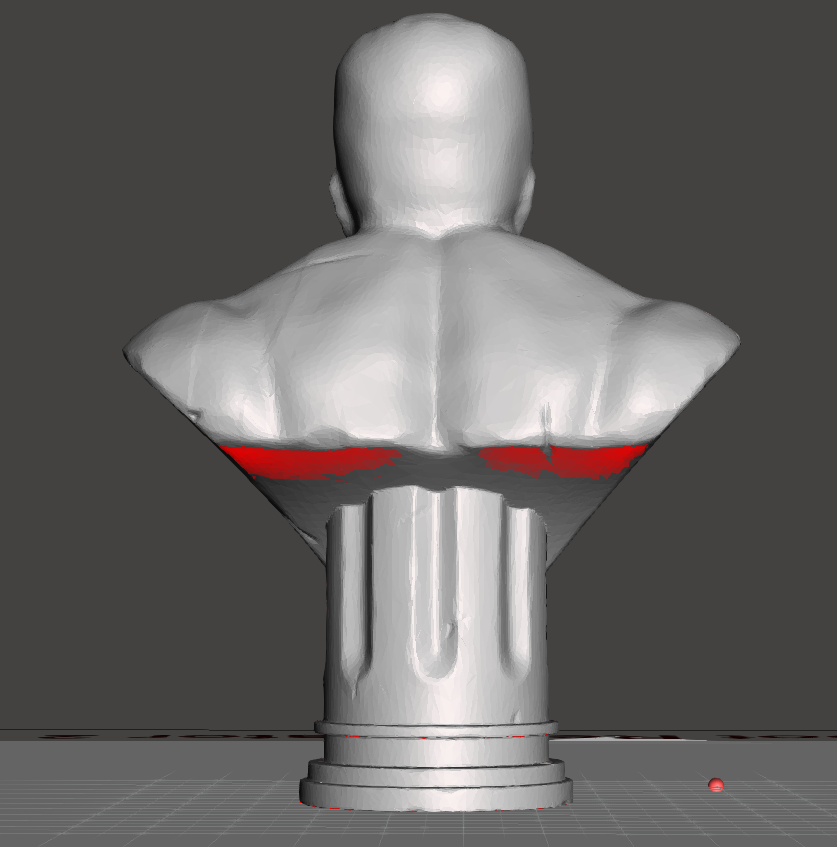
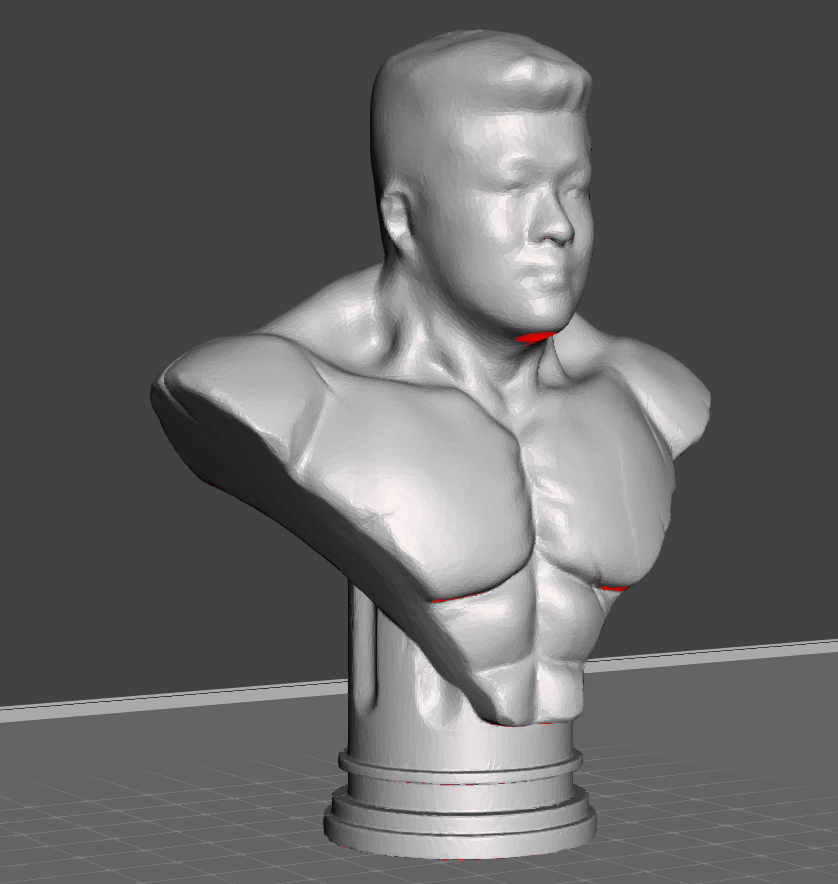
With Meshmixer, there is a great option in “Edit” > “Make Solid” and it generated this for me. Well done! Exactly what I was looking for. Otherwise it prints as hollow.
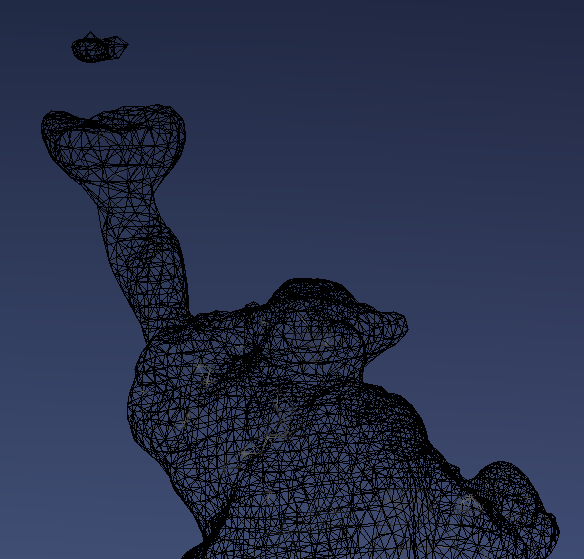
Have to fill in holes here
After some sculpting…

The Meshmixer tools are way more intuitive to use than Blender or anything else for that matter!


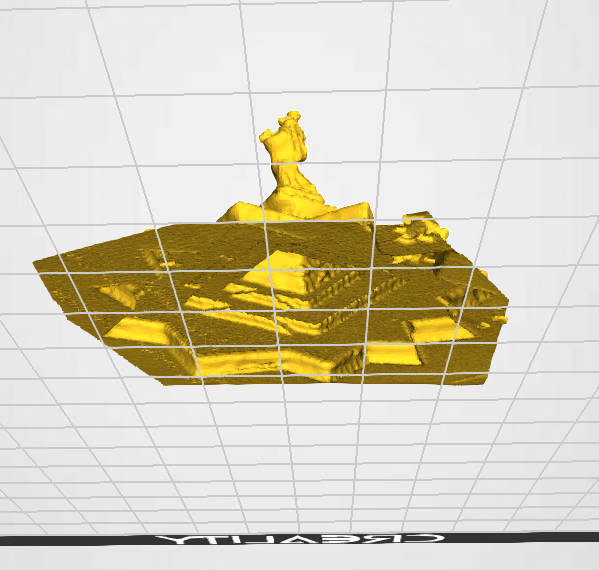
I didn’t notice but the bottom is not completely flat. As I printed this out. There was a power interruption when I used the pressure washer it jumped the breaker. Here’s the result.
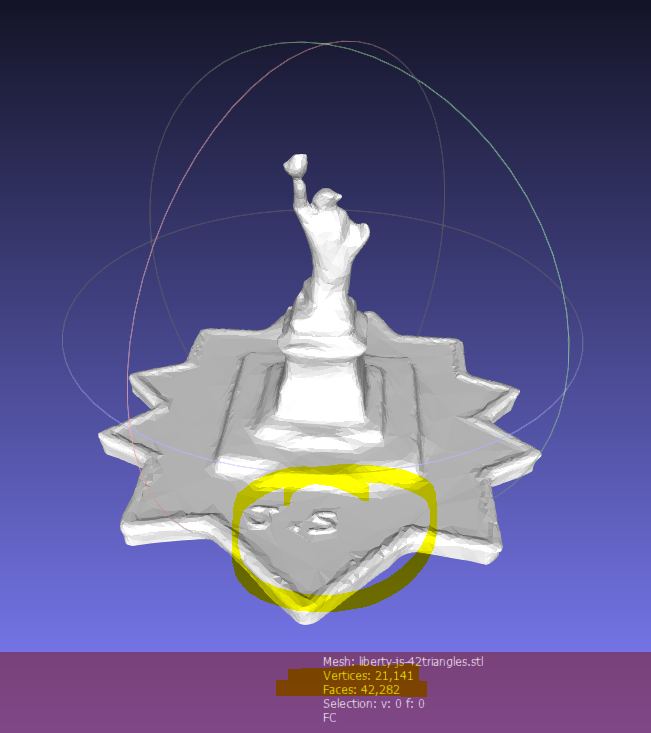
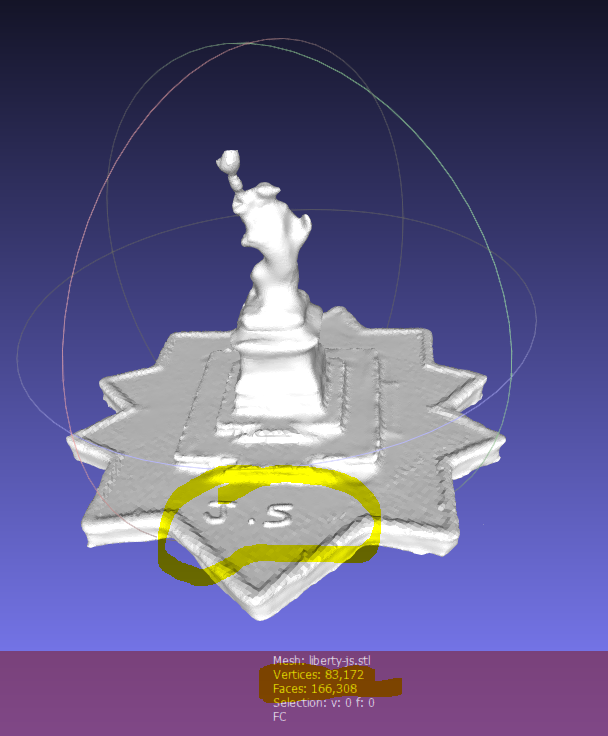
There are also too many polygons with the original. 83,172 Verticies and 166,308 Faces. Using MeshMixer I could reduce verticies to 21,141 and faces 42,282. This lower poly count object should print cleaner too. Notice the surface.





Obviously the wood print failed. But I am happy with the white result!
An octree is a tree data structure in which each internal node has exactly eight children. Octrees are most often used to partition a three-dimensional space by recursively subdividing it into eight octants. Octrees are the three-dimensional analog of quadtrees.
We humans mostly deal with low dimensional data, so we give this type of structure some names:
These are all tree-like data structures, which are very useful for range and nearest neighbor searches.
Space filling curves refers to a class of functions that k dimensional data to 1 dimension.
Meaning a class of functions that can map k-dimensional data into a single number n
f(n_1, n_2, …, n_k) -> n
The caveat is there is a restriction on the number it maps, i.e. n_1, as space filling curves are a fractal functions, it cannot be extended to the reals, but rather to the binary fractions (a subset of the rationals). This lets you get arbitrarily close to any number you want (and cover all the IEEE floating points).
There are certain optimal use cases for each of these.
There are some variants on structures for storing multi-dimensional data.
It’s yet another type of tree.
I won’t go in too much detail because this is out of scope, but in programming there are databases and data stores which can handle large amount of high dimensional data.
First, a distinction. A database can handle complex queries. A data store can be dumber, simple storage format and won’t handle things like transaction for you.
An analogy could be like “database is like an accountant, who you can ask for certain data and operations, such as ‘give me all last year’s data for people with last names in T'”, whereas “data store is like a library and you have to go find and collect that data yourself, but it’s stored in an organized fashion”.